Similarly, the graph of y = x 2 3 is 3 units below the graph of y = x 2 The constant term "c" has the same effect for any value of a and b Parabolas in the vertexform or the ahk form, y = a(x h) 2 k To understand the vertexform of the quadratic equation, let's go back our orginal equation, f(x) = x 2 In this equation, remember This video shows how to use horizontal and vertical shifts together to graph a radical functionThe graph of a quadratic function is a parabola The general form of a quadratic function is f(x) = ax2 bx c where a, b, and c are real numbers and a ≠ 0 The standard form of a quadratic function is f(x) = a(x − h)2 k where a ≠ 0 The vertex (h, k) is located at h = – b 2a, k = f(h) = f

Graph Quadratic Functions Using Transformations Intermediate Algebra
What is the vertex of the graph f(x)=y=a(x-h)2+k
What is the vertex of the graph f(x)=y=a(x-h)2+k-The quadratic function $f(x)=a(xh)^{2}k$ is in standard form (a) The graph of is a parabola with vertex (b) If $a>0,$ the graph of $f$ opens _____ In this case $f(h)=k$ is the _____ value of $f$ (c) If $a F(x) = f(x) − k Table 251 Example 251 Sketch the graph of g(x) = √x 4 Solution Begin with the basic function defined by f(x) = √x and shift the graph up 4 units Answer Figure 253 A horizontal translation 60 is a rigid transformation that shifts a graph left or right relative to the original graph




Vertex Intercept And Standard Form Read Algebra Ck 12 Foundation
Graphing f (x) = a(x − h)2 k CCore ore CConceptoncept Graphing f (x) = a(x − h)2 k The vertex form of a quadratic function is f (x) = a(x − h)2 k, where a ≠ 0 The graph of f (x) = a(x − h)2 k is a translation h units horizontally and k units vertically of the graph of f (x) = ax2 The vertex of the graph of f (x) = a(x − h)2 k is (h, k),The standard form of a quadratic function is f(x) = a(x h) 2 k, a ≠ 0 1 It is always a cupshaped curve 2 It opens upward if a > 0 and opens downward if a < 0 3 The vertex is at (h, k) and the axis of the function is at x = h 4 (h, k) is a maxima if a < 0 and aHere is the graph of y = a ( x − h) 2 k Use the sliders to change the values of a, h, and k so that you are looking at the graph of y = 2 ( x − 1) 2 2 Fill in the following table (The equation for the graph is written below the grid) Equation
Answer to Describe the graph of f(x) = a(xh)^2 k when a = 0 Is the graph the same as that of g(x) = ax^2 bxc when a=0?How to graph a quadratic function using horizontal shiftsMake sure to show the graph INSERT don t ATTACH ;
Learn how to graph in vertex form!C) f(x) = 2(x 3) 2 = 2(x 3)) 2 0 a = 2 , h = 3 and k = 0 The vertex is at (3,0) and it is a minimum point since a is positive Interactive Tutorial Use the html 5 (better viewed using chrome, firefox, IE 9 or above) applet below to explore the graph of a quadratic function in vertex form f(x)=a (xh) 2 k where the coefficients a, h The function p(x) = –8x2 – 64x can be written in vertex form p(x) = a(x – h)2 k, where a =, h =, and k = To graph the function p, reflect the graph of f(x) = x2 across the xaxis, vertically stretch the graph by a factor of 8, shift the graph units, and then shift the graph units
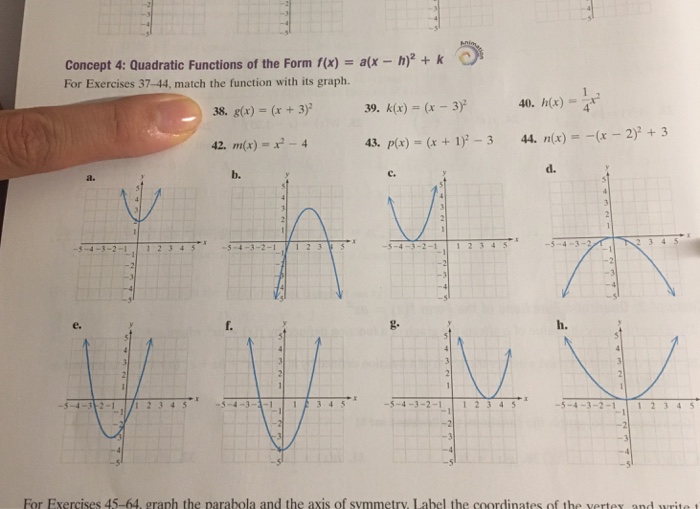



Concept 4 Quadratic Functions Of The Form F X Chegg Com



Why Is It In Vertex Form Of Quadratic Function Y A X H 2 K Getting Value Of H Is Opposite To Its Value Quora
Use the graph of the quadratic function f to write its formula as f(x)= a(x h)2 k f(x)= 6 a 4 G 8 14 112 2 24 62 Get more help from Chegg Solve it with A function is shown f (x) = 16x2 − 1 Choose the equivalent function that best shows the xintercepts on the graph arrow leftThe following applet allows you to select one of 4 parent functions The basic quadratic function f (x) = x^2 The basic cubic function f (x) = x^3 The basic absolute value function f (x) = x The basic square root function y = sqrt (x) In each of these functions, you will investigate what the parameters "a", "h", & "k" will do to the graph the parent function y = f (x) when we graph the function y = a*f (x h) k
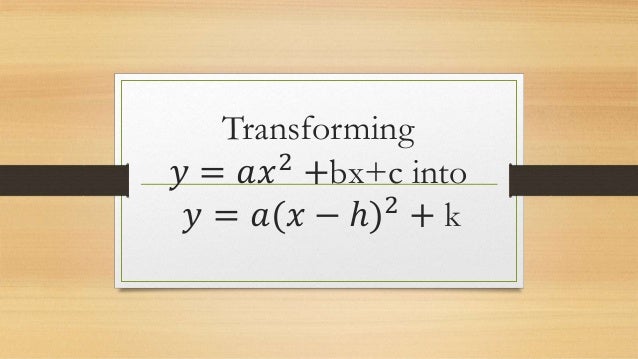



Lesson Effects Of Changing A H And K In The Graph Of Quadratic Functi



Y A X H 2 K Transformations
Learn how to graph just from using the vertex ordered pair and another ordered pair that the graph goes throughThe quadratic function f(x) = a(x − h)2 k is in standard form (a) The graph of f is a parabola with vertex (x, y) = (b) If a >0=a (xh)2k No solutions found Rearrange Rearrange the equation by subtracting what is to the right of the equal sign from both sides of the equation 0 (a* (xh)^2k)=0 Write the quadratic function in the form g (x)= a (xh)^2 k Write the quadratic function in the form g(x) = a(x−h)2 k https//mathstackexchangecom/questions//writethequadraticfunctionintheformgxaxh2k




The Following Graph Of F X X2 Has Been Shifted Into The Form F X X H 2 K Brainly Com
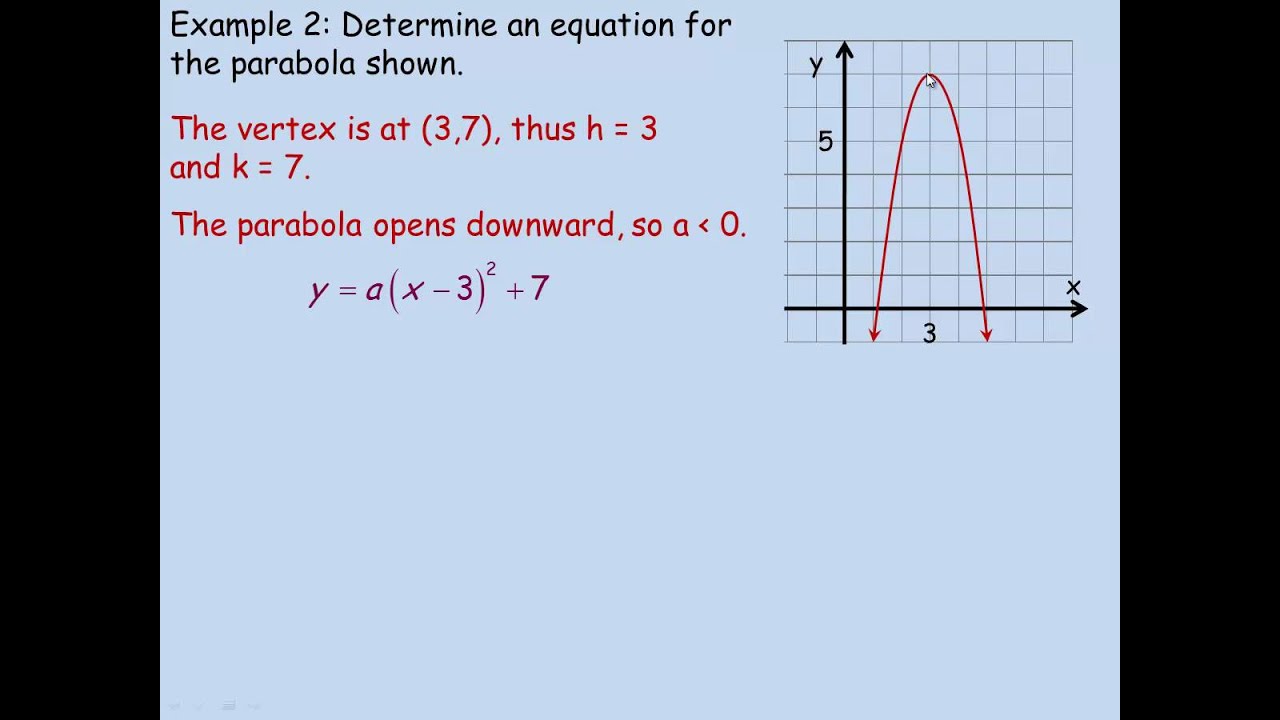



Graphing Y A X H K Youtube
The figure shows a graph of the function $f(x)=x^2$ a) For each of the graphs of quadratic functions below, find values of $a$, $h$, and $k$ so that the function $f(x) = a(x h)^2 k$ has that graph (For example, the graph in the first part corresponds to $a = 1$, $h = 0$, and $k = 0$)This video shows how to use vertex form ie y = a(x h)² k to graph a parabola or use it to write an equation from a graph This lesson was created foCalculus Q&A Library he quadratic function f(x)=a(xh)^2k is in standard form (a) The graph of f is a parabola with vertex (x, y) = (_____) (b) If a > 0, the graph of f opens upward/downward In this case f(h) = k is the (maximum/minimum)value of f (c) If a < 0, the graph of f opens (upward/downward)




Quadratic Function Wikipedia



Illustrative Mathematics
Graph the function using transformations Graph a quadratic function in the vertex form f(x) = a(x − h)2 k using properties Rewrite the function in f(x) = a(x − h)2 k form Determine whether the parabola opens upward, a > 0, or downward, a < 0 Find the axis of symmetry, x = hQuestion Write the quadratic function in the form f(x)=a(xh)^2k Then, give the vertex of its graph f(x)=−2x^212x any help greatly appreciated Answer byA quadratic function f in vertex form is written as f (x) = a (x h) 2 k where h and k are the x and y coordinates respectively of the vertex (minimum or maximum) point of the graph The graph of of f is a parabola with the vertical line x = h as an axis of symmetry



Sections 6 And 8 Quadratics Part 2 And Polynomial Functions




Quadratic Functions



0 件のコメント:
コメントを投稿